Difference between revisions of "SWF e-book"
From Organic Design wiki
m |
(will fix later, document runtime state of problem for now) |
||
| Line 10: | Line 10: | ||
*Line '''D''' is perpendicular to line '''C''' and passes through point '''Z''' which is the midpoint of line '''C''' | *Line '''D''' is perpendicular to line '''C''' and passes through point '''Z''' which is the midpoint of line '''C''' | ||
*Source code is [[SWF e-book.as]] | *Source code is [[SWF e-book.as]] | ||
| + | |||
| + | = Current bug = | ||
| + | The current problem which I'll check out a bit more soon is that at a certain point moving outside the top-right of the page causes a rendering problem, it appears as a large triagular page where it should actually exit without folding at all in those scenarios. Here's a table showing the values of points P,Q,R and co-ordinates x,y and dx,dy for three cases of movement near the problem area. The first column is the working case, the second is the triangle problem which has its left most point in the books top-center, and the last column is the triangle with left point far off the left side. | ||
| + | {| border cellspacing=0 cellpadding=2 | ||
| + | |- | ||
| + | |'''P''' | ||
| + | |(0,0) | ||
| + | |(0,0) | ||
| + | |(∞,0) | ||
| + | |- | ||
| + | |'''Q''' | ||
| + | |(250,5) | ||
| + | |(250,300) | ||
| + | |(250,300) | ||
| + | |- | ||
| + | |'''R''' | ||
| + | |(250,5) | ||
| + | |(250,300) | ||
| + | |(250,300) | ||
| + | |- | ||
| + | |''x,y'' | ||
| + | |(250,10) | ||
| + | |(0,<1) | ||
| + | |(250,0) | ||
| + | |- | ||
| + | |''dx,dy'' | ||
| + | |(0,10) | ||
| + | |(250,<1) | ||
| + | |(0,0) | ||
| + | |} | ||
Revision as of 02:31, 23 December 2006
SWF e-book.xml|set-publish.xml|swf.php
| SWF e-book.xml|swf.php|nowiki.php |
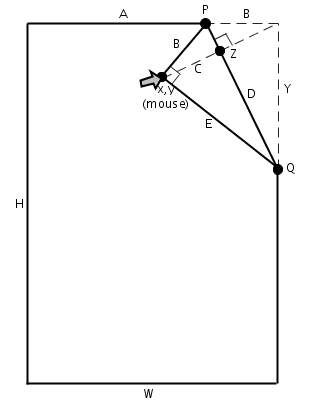
- The foundation of the effects can be built from resolving points P and Q
- P and Q are obtained from the intersections of the top and right page edges with line D
- Line D is perpendicular to line C and passes through point Z which is the midpoint of line C
- Source code is SWF e-book.as
Current bug
The current problem which I'll check out a bit more soon is that at a certain point moving outside the top-right of the page causes a rendering problem, it appears as a large triagular page where it should actually exit without folding at all in those scenarios. Here's a table showing the values of points P,Q,R and co-ordinates x,y and dx,dy for three cases of movement near the problem area. The first column is the working case, the second is the triangle problem which has its left most point in the books top-center, and the last column is the triangle with left point far off the left side.
| P | (0,0) | (0,0) | (∞,0) |
| Q | (250,5) | (250,300) | (250,300) |
| R | (250,5) | (250,300) | (250,300) |
| x,y | (250,10) | (0,<1) | (250,0) |
| dx,dy | (0,10) | (250,<1) | (0,0) |







